Gravitational deflection of light
On one of the fundamental consequences of general relativity: the deflection of light by gravity
An article by Steven S. Shapiro, Irwin I. Shapiro
Theories of the deflection of light by mass date back at least to the late 18th century. At that time, the Reverend John Michell, an English clergyman and natural philosopher, reasoned that were the Sun sufficiently massive, light could not escape from its surface. The pioneer of a mathematical description of gravity, Sir Isaac Newton, apparently wrote nothing about the effect of mass on the path of light rays, other than to note at the end of his treatise, “Opticks,” published in 1704, that light particles should be affected by gravity in the same way as is ordinary matter.
The first calculation of the deflection of light by mass was published by the German astronomer Johann Georg von Soldner in 1801. Soldner showed that rays from a distant star skimming the Sun’s surface would be deflected through an angle of about 0.9 seconds of arc, or one quarter of a thousandth of a degree. This angle corresponds to the apparent diameter of a compact disc (CD) viewed from a distance of about 30 kilometres (nearly 20 miles). Soldner’s calculations were based on Newton’s laws of motion and gravitation, and the assumption that light behaves like very fast moving particles. As far as we know, neither Soldner nor later astronomers attempted to verify this prediction, and for good reason: Such an attempt would have been far beyond the capability of early 19th century astronomical instruments.
Light deflection in general relativity
Over a century later, in the early 20th century, Einstein developed his theory of general relativity. Einstein calculated that the deflection predicted by his theory would be twice the Newtonian value.
The following image shows the deflection of light rays that pass close to a spherical mass. To make the effect visible, this mass was chosen to have the same value as the Sun’s but to have a diameter five thousand times smaller (i.e., a density 125 billion times larger) than the Sun’s.
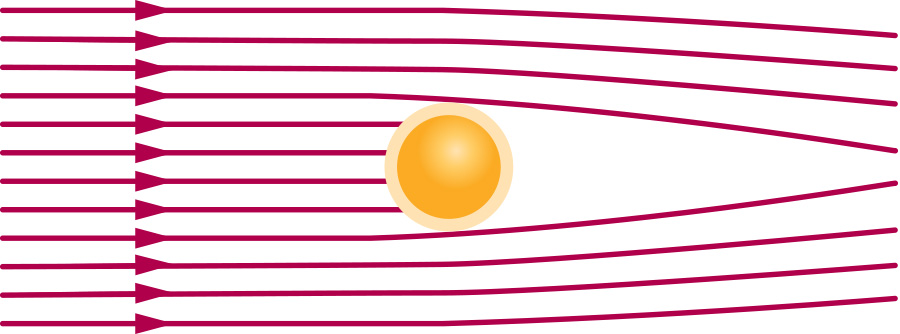
According to general relativity, a light ray arriving from the left would be bent inwards such that its apparent direction of origin, when viewed from the right, would differ by an angle (α, the deflection angle; see diagram below) whose size is inversely proportional to the distance (d) of the closest approach of the ray path to the center of mass.
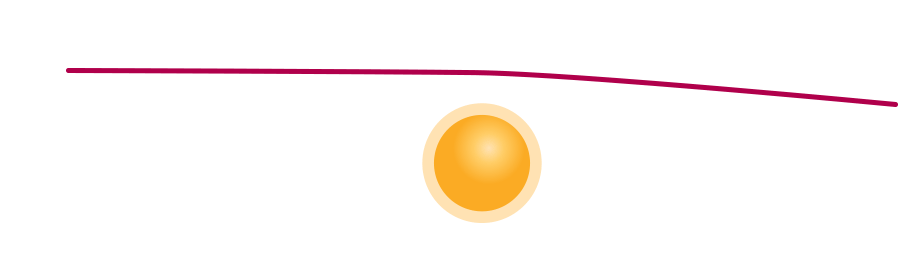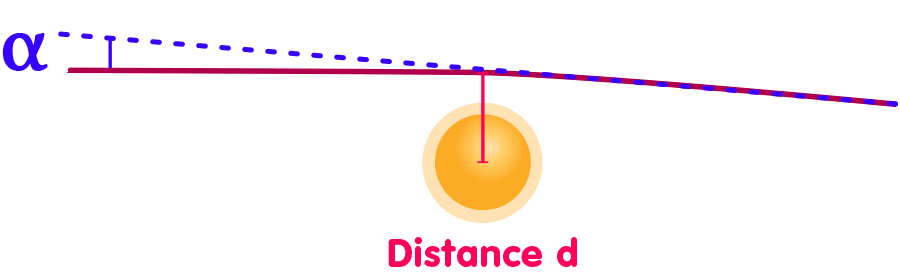
A graph of α as a function of d for this compact spherical mass is shown below:
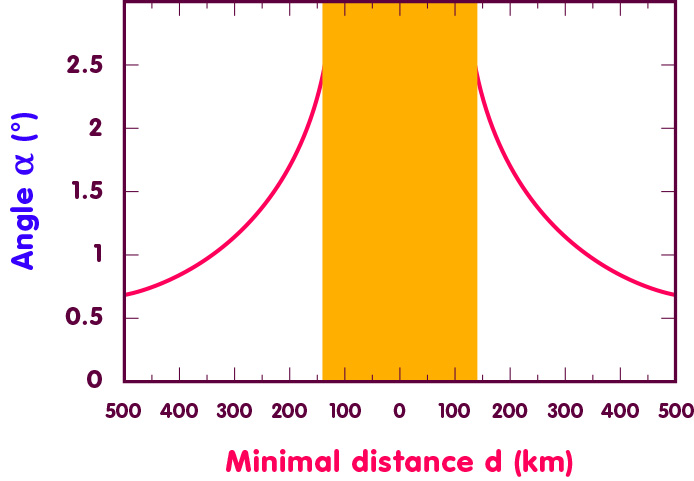
The yellow area in the center indicates the spatial extent of the spherical mass. The curves to each side show the dependence of the deflection angle α on the distance d. The deflection angle is largest when the light rays pass closest to the mass; α becomes smaller as d becomes larger. For the Sun, the curves look similar, but the predicted value of α is five thousand times smaller for rays that skim the surface of the Sun than for rays that skim the surface of this “pseudo” Sun.
Shifting positions
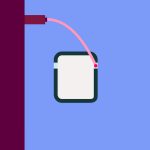
An observer on the Earth can detect the deflection by the Sun of the light from a (distant) star by the change with time of year in the star’s apparent position in the sky. The following animation shows light from a star on the left and an observer on the right, and the change in apparent position of the star caused by the presence of a mass in between:
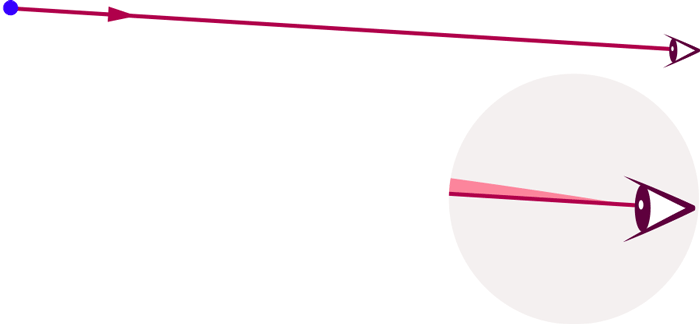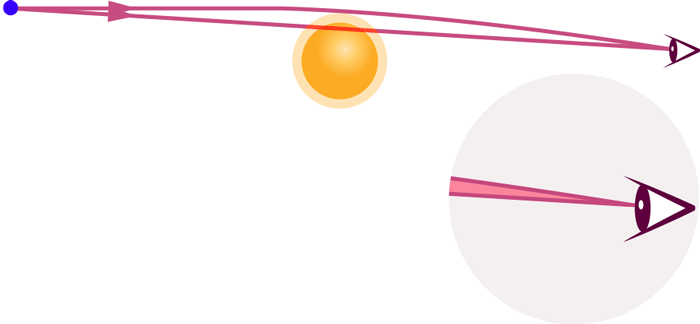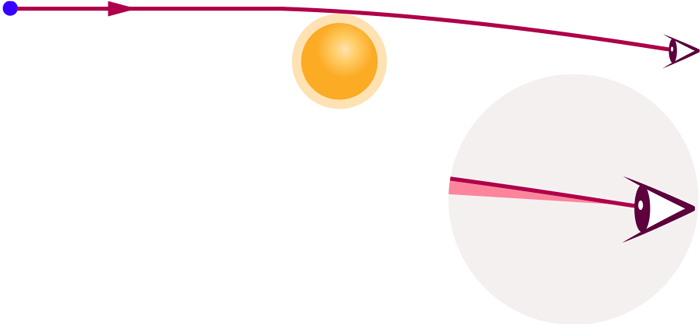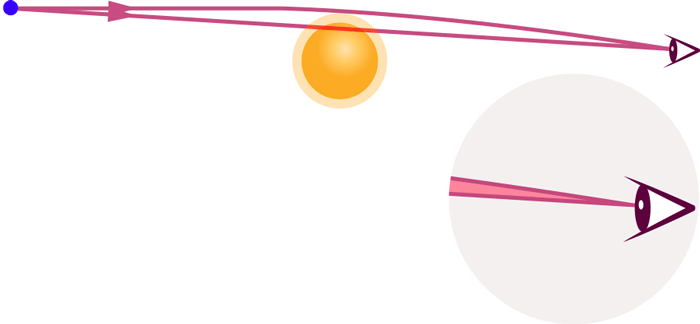
The inset shows a magnified view of how the light rays reach the observer. In the absence of a mass, the light follows a straight line from the star to the observer. In the presence of the mass, the light ray is bent, and the light reaches the observer from a slightly different direction. This direction defines a star’s apparent position in the sky.
Such shifts in position – although far smaller than in the images above – should be visible to a properly equipped optical observer on the Earth for a star near the Sun’s path in the sky. However, under ordinary conditions sunlight causes the atmosphere to be so bright that it is not feasible to observe from the Earth with optical telescopes any star whose light passes near the Sun. For the first tests of Einstein’s predictions, astronomers therefore used solar eclipses, occasions on which the moon is between the Earth and the Sun, and blocks all sunlight from reaching the vicinity of the Earth and brightening its atmosphere.
Measuring light deflection
1919 saw the first successful attempt to measure the gravitational deflection of light. Two British expeditions were organized and sponsored by the Royal Astronomical Society and the Royal Society. Each of the two groups took photographs of a region of the sky centered on the Sun during the May 1919 total solar eclipse and compared the positions of the photographed stars with those of the same stars photographed from the same locations in July 1919 when the Sun was far from that region of the sky. The results showed that light was deflected, and also that this deflection was consistent with general relativity but not with “Newtonian” physics. The subsequent publicity catapulted Einstein to world fame, and led to his having the only ticker-tape parade ever held for a scientist on Broadway in New York City.
With repetitions of eclipse measurements over the next half century, astronomers were able to improve on the accuracy of these first results by only about a factor two, yielding a confirmation of general relativity to within about ten percent. The breakthrough came in 1967 with the realization that simultaneous measurements with a set of radio telescopes (especially, “Very Long Baseline Interferometry”) could be used to measure light deflection with much greater accuracy.
In addition to providing the means to test general relativity to high accuracy, the fact that mass deflects light has been a great boon to studies of the universe. Masses acting as gravitational lenses have now become a standard tool of astronomy. They allow astronomers to infer the masses of cosmic objects, and the structure and size scale of the universe (with some caveats). Through their magnifying effect, gravitational lenses have also been used to observe the properties of very distant galaxies and quasars, as well as to search for planets around distant stars.
Further Information
For the relativistic ideas behind this spotlight topic, check out Elementary Einstein, especially the chapter on General Relativity.
Related Spotlights on relativity can be found in the section General Relativity.
Colophon
is a physics professor at Lesley University, Cambridge (USA).
is the Timken University Professor at Harvard University and a Senior Scientist of the Smithsonian Institution.
Citation
Cite this article as:
Steven S. Shapiro, Irwin I. Shapiro, “Gravitational deflection of light” in: Einstein Online Band 04 (2010), 03-1003