The dialectic of relativity

How relativity can reconcile statements that, at first glance, appear to be contradictory

An article by Markus Pössel

When first encountered, some of the statements of special relativity sound downright paradoxical. However, in most cases the suspected paradox can be resolved within a framework of a “dialectic of relativity”. (Here, the term “dialectic”, which is borrowed from philosophy, that describes how a thesis and a seemingly contradictory anti-thesis can be reconciled by a higher truth.)

Relativity at the breakfast table

The basic concept of relativity is something we know from everyday life. The prime example is relative position: Imagine me sitting at the breakfast table. I claim that my teacup is in front of the teapot, while my wife claims that it is behind the teapot. Taken at face value, those are contradictory statements – how can one and the same cup, at one and the same time, be in front and behind the teapot?

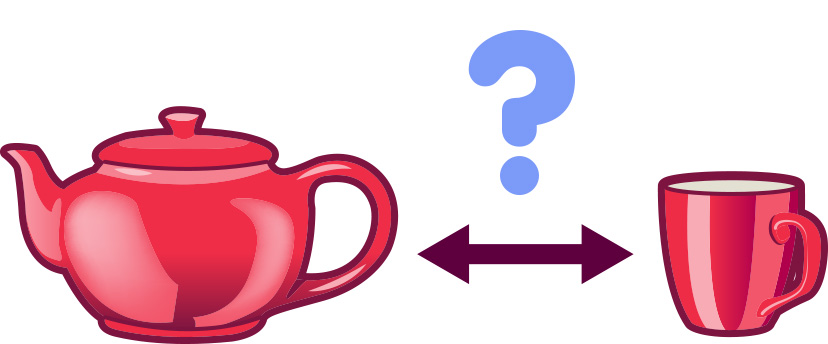
The apparent contradiction is resolved as soon as you realize the relativity of “behind” and “in front of”. My wife and I are sitting on opposite sides of the table, facing each other. If an object A is “in front of” another object B from my point of view, it is “behind” that object as seen from my wife’s perspective. The seemingly contradictory “in front of” and “behind” refer to two different reference frames. Once this is properly taken into account, the apparent contradiction goes away.

The same technique (“for each statement, carefully determine the reference frame”) for the resolution of apparent contradiction can be put to work where special relativity is concerned.

The dialectic of time dilation

As an example, consider time dilation: Imagine that I am sitting in a space station, drifting through space. (In the jargon of special relativity, my space station is an inertial reference frame.) Assume that a second space station, as free as my own (another inertial reference frame), passes my own station at high speed.

Due to the phenomenon of time dilation, I will observe that clocks on the other space station go more slowly than my own clocks. And not only the clocks: all processes appear to take more time on the moving station; the station’s inhabitants age more slowly, for instance.

On the other hand, in special relativity, all freely drifting space stations (more precisely: all inertial reference frames) are on an equal footing. That is the content of the relativity principle: For all such stations, the laws of physics are the same. From the point of view of an observer aboard that other space station, my own station is passing by at high speed, and the consequences are the same: for such an observer, a clock moving at high speed – and from his point of view, my own clock falls under that description – goes more slowly than his own clocks.

We have here an apparent contradiction: how can both statements be true? How can my own clocks be slower than those of the other space station, and the other station’s clocks slower than mine?

The need for comparing clocks twice

Just as at the breakfast table, the apparent contradiction can be resolved if the relativity of the statements in question is taken into account. The key point: If you want to find out which of two clocks runs faster, it is not enough to compare them a single time; you need to compare them at least two times. For example, suppose that at the first comparison, the blue and the red clock show the same time:

However, that means nothing at all. It could be that the blue clock is at a standstill and shows 12:30 all the time, while we have caught the red clock, which is running normally, in the single minute it shows 12:30 as well. Only the second comparison can tell:

At least in the time interval in question, the red clock has indeed run faster than the blue one – on the red clock, 15 minutes have passed, compared with 13 minutes on the blue clock.

However, with clocks that are moving with constant speed relative to each other, there is only a single moment at which they are at the same location and can be compared directly. For the second comparison, slightly later, the clocks will necessarily be a bit apart, at different locations; their movement relative to each other makes this inevitable. That is where the notion of simultaneity comes into play: comparing the two clocks is the same as determining whether or not two events (such as “the blue clock shows 12:45” and “the red clock shows 12:45”) happen simultaneously or not.

In Einstein’s theory, there is a definition of simultaneity that employs light signals going back and forth (cf. The definition of “now”). However, simultaneity thus defined is relative. Observers that are in motion relative to each other (and that employ Einstein’s definition of simultaneity) will generally end up with different results: events that one observer judges to be simultaneous will not necessarily be simultaneous for the other.

This insight allows relativity to escape the apparent contradiction that one and the same clock is both slower and faster than another clock. When I come to the conclusion that the clocks in the other space-station are slower, I rely on my own concept of simultaneity for the comparison. The other observer, coming to the conclusion that my clocks run slower, relies on his own concept of simultaneity. (A simple geometric analogy to this is explored in the spotlight topic Time dilation on the road.)

Just as in the case of “in front of” and “behind”, the two statements “slower” and “faster” refer to different frames of reference. There are several similar situations in special relativity – contradictory at first glance, but readily resolved once the different frames of reference, and especially the different concepts of simultaneity, are taken into account.

Further Information