The elevator, the rocket, and gravity: the equivalence principle
Information about the principle that Einstein took as a starting point for developing his general theory of relativity
An article by Markus Pössel, AEI
By 1905, Albert Einstein had created a new framework for the laws of physics – his special theory of relativity. However, one facet of physics appeared to be incompatible with his new ideas: the gravitational force as described by Newton’s law of gravity. Special relativity provides a new framework for physics only when gravity is excluded. Years later, Einstein managed to unify gravity and his relativistic ideas of space and time. The result was another revolutionary new theory, general relativity.
Einstein’s first step towards that theory was the realization that, even in a gravitational field, there are reference frames in which gravity is nearly absent; in consequence, physics is governed by the laws of gravity-free special relativity – at least to a certain approximation, and only if one confines any observations to a sufficiently small region of space and time. This follows from what Einstein formulated as his equivalence principle which, in turn, is inspired by the consequences of free fall.
In defining the equivalence principle, there are certain subtleties. In the paragraph above, they are merely hinted at (“approximately”, “small region”). Let us ignore them a bit further and start with a simplified version of the principle, beginning with a simple set of thought experiments.
Equivalence principle: A simplified definition
Imagine you are in an elevator or, more precisely, in what looks like an elevator cabin from the inside, and that you are isolated from the outside world. If you pick up an object and let it drop, it falls down to the floor, in exactly the way you would expecte given your experiences here on Earth. Does that mean the elevator is indeed situated in a gravitational field like that of the Earth – as shown in the following illustration?

It ain’t necessarily so. Theoretically, you could be in deep space, far away from all significant mass concentrations and their gravitational influence. The room you are in could be a cabin aboard a rocket – as long as the rocket engines work at exactly the right rate to accelerate the rocket at 9.81 metres per square second. This is sketched in the following illustration:
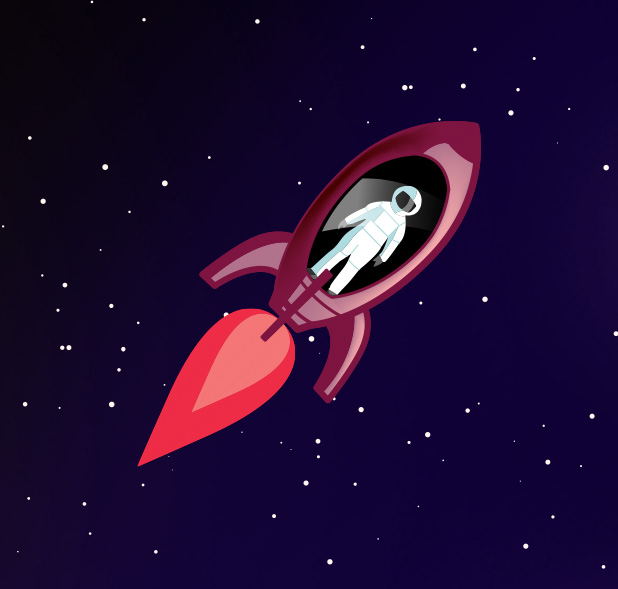
In such a situation, if you let go of an object, the cabin floor would accelerate towards that object at 9.81 metres per square second – exactly the same acceleration of free fall here on earth. From your vantage point as an observer standing on the cabin floor, you cannot distinguish between the two situations: are those objects falling towards a massive body like the earth? Or is the cabin floor accelerating towards them?
(Addendum April 26, 2014: What happens with objects that do not fall down in a gravitational field on Earth, but rise up – such as a helium balloon? As this YouTube video shows, this situation obeys the equivalence principle, as well. In both cases, the air molecules that move downward/toward the cabin floor push the balloon upward – in the opposite direction of the gravitational field or in the same direction as the space ship’s acceleration, respectively.)
Weightlessness poses a similar problem. Imagine you’re floating freely inside the elevator. Around you, other objects are floating, as well, and you feel totally weightless. Does that mean you are far away from all gravitational influences, far away from all stars, planets and other massive bodies, somewhere in deep space?
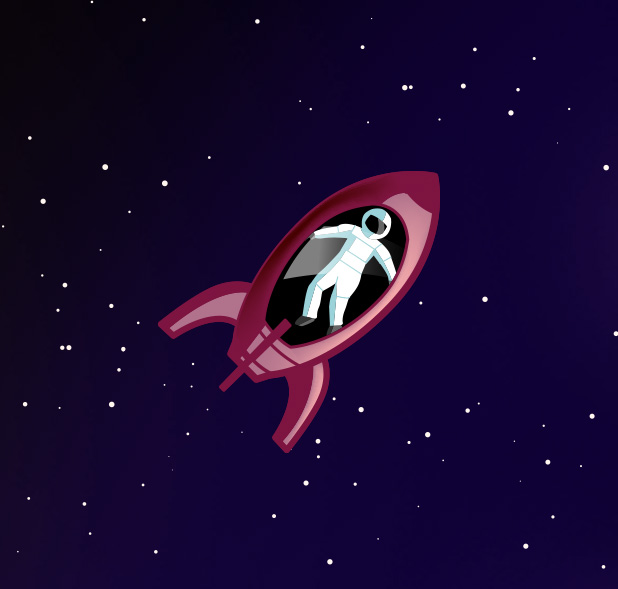
Again, you cannot be sure. Alternatively, you and the elevator could be in the gravitational field of a mass, for instance that of the earth, as long as the elevator was in free fall. In that case you, everything else within the elevator and the elevator itself would all be accelerated at exactly the same rate so that, inside, no influence of gravity could be detected. Relative to the elevator, all those objects faithfully keep their relative positions (or move at a constant speed), just as they would in a gravity-free region of space. You, as the elevator’s passenger, would feel weightless – after all, in an ordinary situation here on earth, you feel your weight as gravity pulls your body down, pressing whatever part of it carries your weight onto the floor. In the falling elevator, both your body and the floor fall in parallel, at the same rate:
This is the kind of weightlessness experienced, for instance, by astronauts on the International Space Station (ISS). After all, it is not as if space station and crew had escaped the earth’s gravitational field – at that particular altitude, the pull of the gravitational force is still 90 per cent as strong as on the earth’s surface. The astronauts’ weigtlessness is due to the fact that, along with their station, they are in free fall. Not in the kind of free fall that takes them directly towards the earth, but in free fall that takes them around the earth – in earth orbit.
Thus, inside an elevator, we cannot decide whether or not we are in a gravitational field or not. Whether or not objects accelerate towards the floor is a matter of reference frame: Even in a gravity-free region of space, objects fall towards the floor if the room we are in is being accelerated. Conversely, even in a gravitational field, objects drift weightlessly through space, as long as the elevator is in free fall.
Einstein became convinced that this inability to distinguish a region with a gravitational field from one without was not just restricted to observations of falling bodies. He postulated that it holds true for any physical measurements at all: No experiment, no clever exploitation of the laws of physics, he claimed, can tell us whether we are in free space or in a gravitational field. This statement is called the equivalence principle. One of the consequences: In a reference frame that is in free fall, the laws of physics are the same as if there were no gravity at all – the laws of physics are those of special relativity!
Tidal forces, and a more precise definition
So far, so simple. Too simple, in fact, in several respects. Strictly speaking, all that was said about the equivalence of gravity and acceleration is true only for gravitational fields that are strictly homogeneous. Only in homogeneous gravitational fields are all bodies – per definition – accelerated in exactly the same way, namely in exactly the same direction and at exactly the same rate; as a result, it is indeed true that a researcher inside a cabin cannot distinguish acceleration from gravity. But real gravitational fields are always to a certain extent inhomogeneous.
Take, for example, the gravitational field of the earth. True, here on the surface, looking at experiments which take up only a very, very small fraction f the total surface area of the earth, the gravitational field is, to good approximation, homogeneous: all objects fall to the floor along parallel paths, in the same direction (“down”) and with the same acceleration (at least as long as the effects of air friction can be neglected). But if we look closer, the situation is a bit more complex. Here is an example where the deviations from homogeneity are clearly visible – a truly gigantic elevator which contains two spheres, all falling towards the earth:
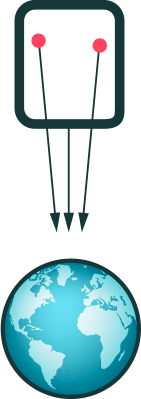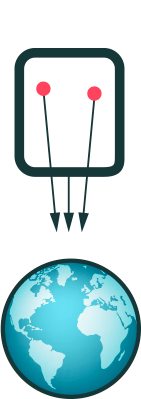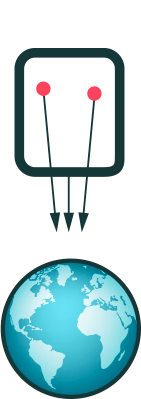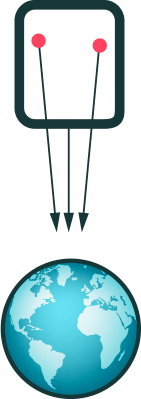
This extreme example shows clearly: the elevator and the spheres do not fall in parallel. Instead, they fall towards one and the same point, the earth’s centre of gravity. And while an observer inside the elevator does not see the common downward component of the fall, he or she will notice that the two spheres move slightly closer together.
This is what is called a tidal effect. Tidal effects are what tells a freely falling observer that he is in an inhomogeneous gravitational field, and thus definitely not in gravity-free space. Thus, a more precise formulation of the equivalence principle states that in any freely falling reference frame, the laws of physics are the same as in special relativity, as long as tidal effects can be neglected.
One can, in fact, be more specific as to how tidal effects can be kept small: first of all, by confining all observations to a small region of space: in the animation above, the effects are clearly visible because the distance between the two spheres is not that much smaller than their distance to the earth. For someone here on earth dropping two objects a mere few metres apart, the effect will be virtually undetectable. On the other hand, if you watch merely a brief excerpt from the above animation, you will hardly see the two spheres move towards each other.
Realizing that what matters are the size of the region, and the duration of our observations, we are led to a formulation in which the equivalence principle is not just a useful approximation, but exactly true: Within an infinitely small (“infinitesimal”) spacetime region, one can always find a reference frame – an infinitely small elevator cabin, observed over an infinitely brief period of time – in which the laws of physics are the same as in special relativity. By choosing a suitably small elevator and a suitably brief period of observation, one can keep the difference between the laws of physics in that cabin and those of special relativity arbitrarily small.
Further Information
This spotlight topic complements the information given in the section General relativity of Elementary Einstein.
The path from the equivalence principle to Einstein’s general relativity is traced in the spotlight text Gravity: From weightlessness to curvature. Related spotlights on relativity can be found in the category General relativity. The relationship between the principle and one of the classic effects predicted by general relativity is examined in The equivalence principle and light deflection.
Colophon
is the managing scientist at Haus der Astronomie, the Center for Astronomy Education and Outreach in Heidelberg, and senior outreach scientist at the Max Planck Institute for Astronomy. He initiated Einstein Online.
Citation
Cite this article as:
Markus Pössel, AEI, “The elevator, the rocket, and gravity: the equivalence principle” in: Einstein Online Band 01 (2005), 01-1009