Actors on a changing stage: quantum gravity and background independence

The principle of background independence – space and time are no fixed structure, but take part in the dynamical evolution of the world – and its consequences for the problem of quantum gravity

An article by Lee Smolin

Presently, there are a number of approaches to the problem of finding a theory of quantum gravity, the most prominent being string theory and loop quantum gravity. A key difference between these approaches is how they deal with the fundamental question of what physicists call background independence.

Are space and time fixed background structures? The history of this question goes back to the ancient Greeks, but the issues of interest to modern physicists came into focus only with the advent of classical mechanics as formulated by Isaac Newton.

Space and time as a stage

In Newton’s cosmos, space and time provide a fixed, immutable and eternal background, with respect to which particles move. Space and time are a stage. Like actors on that stage, particles move, exert forces onto each other and generally act out the drama of dynamics, while the stage itself does not change. This is sketched in the following illustration. There, we see objects, pictured as geometrical shapes, but there is also a fixed background structure, space, which is just as real, pictured here as a lattice of intersecting lines:

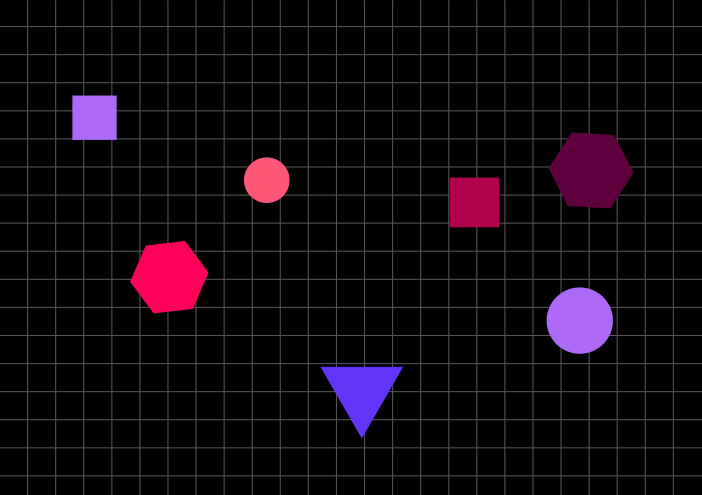
Newton’s cosmos: space and time provide a fixed, immutable and eternal background

Newton’s view of the universe is manifestly background dependent – whether or not a particle is moving or at rest is determined in relation to Newton’s absolute space and time.

Relationism

But even in Newton’s day, there were philosophers like Gottfried Wilhelm Leibniz who took a different stance. In modern parlance, theirs is a relationist point of view. For a relationist, there is no background of absolute space and time. The fundamental properties of the elementary entities consist entirely in relationships between those elementary entities. Time is nothing but changes in these relationships, and consists of nothing but the ordering of these changes. In the relationist version of the above illustration, only the objects, pictured as geometrical shapes, are real. The lattice has no independent existence. Even without the lattice, it does make sense to talk about the distance between two given objects. But it does not make sense to talk about the distance between two space points where there are no objects at all:

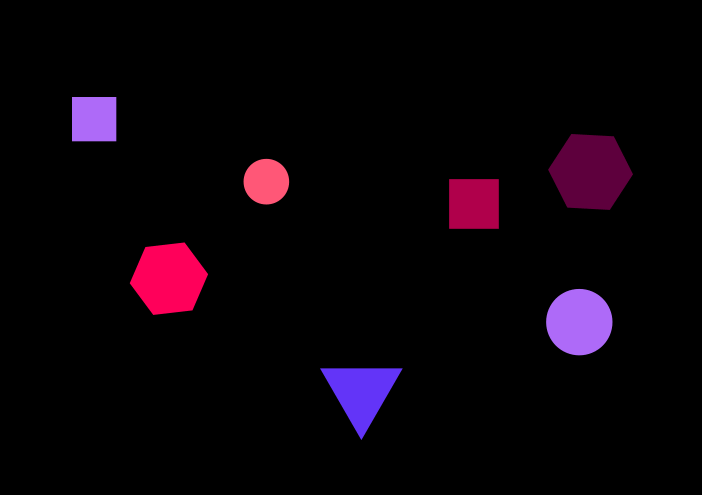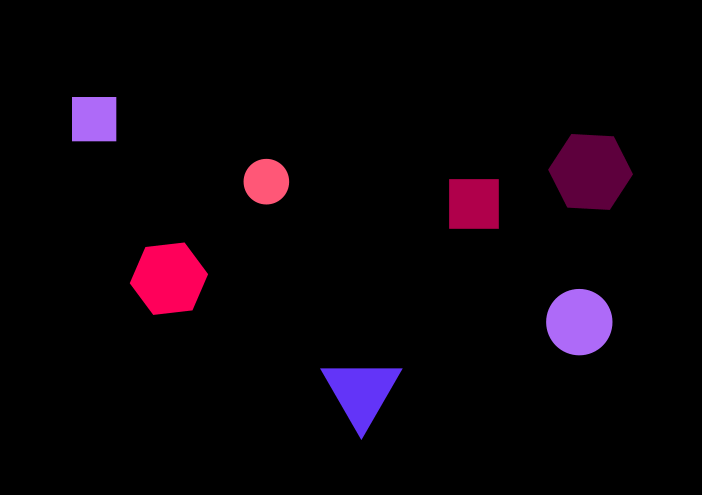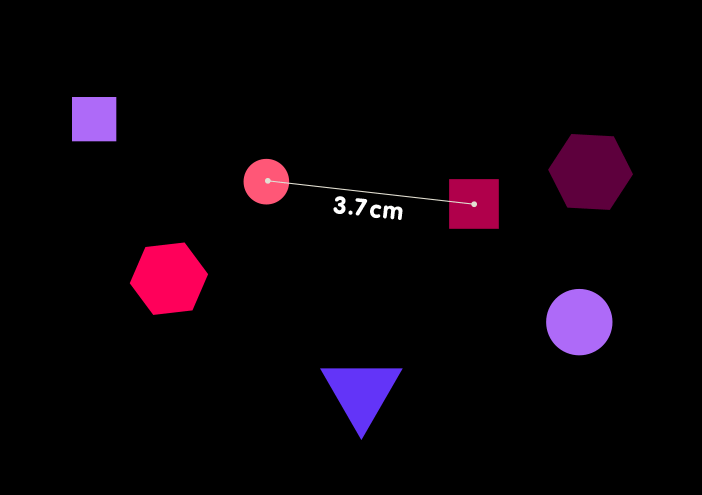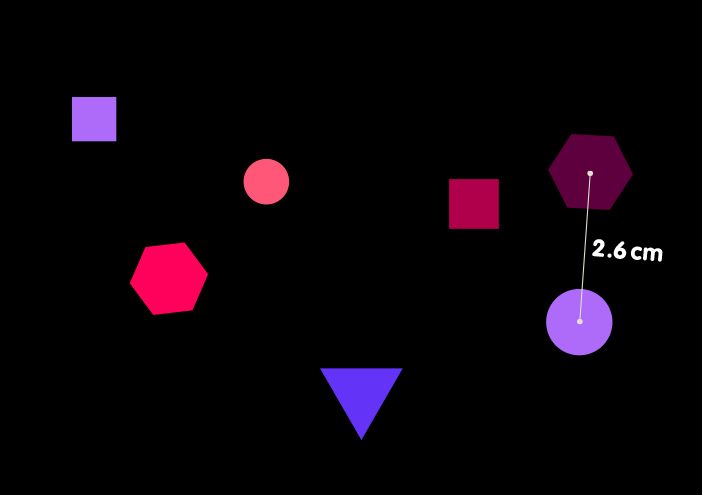
A relational world without spatial background

One of the fundamental discoveries of Einstein is that in our universe, there is indeed no fixed spacetime background. In Einstein’s theory of general relativity, which replaces Newton’s theory of mechanics and the gravitational force, the geometry of spacetime is not fixed. Instead it is an evolving, dynamical quantity. Physicists intent on describing our universe cannot just assume a given geometry. Instead, geometry is something that needs to be determined by solving certain equations that include all the effects of matter and energy.

A crucial aspect of Einstein’s dynamic geometry are gravitational waves, wave-like, travelling distortions of space geometry. Thus, experimental observations that energy leaks from binary pulsars such as PSR1913+16 in the form of gravitational waves at the exact rate predicted by general relativity tells us that there is no more a fixed background of spacetime geometry than there are fixed crystal spheres holding the planets up.

Equivalent lattices

Closely related to background independence is another basic ingredient of general relativity, known by the imposing name diffeomorphism invariance. It concerns the coordinates physicists use to describe space and time. The principle of diffeomorphism invariance implies that, unlike in theories prior to general relativity, there are no additional structures in physics that allow us to distinguish preferred coordinate systems. As far as the laws of physics are concerned, no coordinate system is better than another, and one is free to choose. In terms of the simplified illustration above, there are infinitely many ways to choose a lattice – a few examples are shown here:

The same situation, described with the help of different coordinates

By diffeomorphism invariance, the fact that a point lies on the y=9 line of one lattice, or on the wavy x=3 curve of another, or at the intersection of y=5 and x=2 in the third, is of no importance to physics. A point in space is defined only by the presence of an object, say, the blue triangle, not by its location according to some special set of coordinates. Or, to go back from the simplified illustration to general relativity: A point in spacetime (an event) is defined only by what physically happens at it, not by its location according to some special set of coordinates. The physics does not depend on what lattice you choose.

With its dynamical geometry and the inability of pinning geometry down in terms of a rigid lattice (diffeomorphism invariance), general relativity is at least a partly relational theory. In fact, the relational/absolute debate is the basic historical and philosophical context for Einstein’s work.

The search for quantum gravity

In the search for a theory of quantum gravity, it makes sense to adopt a similar relational strategy: To find the fundamental theory of nature, we should seek to make progress by identifying the background structures in our theories and removing them, replacing them with relations which evolve subject to dynamical law. This amounts to saying we believe that the triumph that general relativity represented for the relational point of view is final and will not be reversed.

Of the approaches to quantum gravity already mentioned, the current incarnations of string theory are formulated in a manifestly background-dependent way. In each of the different models of string theory, the fundamental constituents, the strings, are defined as moving in a fixed background of space and time. This may be a useful approximation, but it cannot be the formulation of a fundamental theory of quantum gravity. Only recently, in the 1990s, has the idea of background independence gained some prominence in the string theory community. Back then, string theorists postulated that all the different string theories so far discovered plus an infinite number of so-far-undiscovered theories are but approximations to a single, unified theory that has been called M theory. There is no general agreement yet as to what the fundamental principles of M theory are or what mathematical form it takes. The idea is that M theory, if it exists, would be background-independent, and that all the different background-dependent string theories would be special cases of the more general theory. However, to reach that state of affairs, it seems that string theorists still have a long way to go.

In contrast, loop quantum gravity is indeed widely background independent. It gives us a detailed description of quantum spatial and spacetime geometry, with many encouraging results. The quantum geometry is formulated using beautiful relational structures such as connected networks with nodes and connections (compare the page Loop quantum gravity in the section Relativity and the quantum of Elementary Einstein as well as the spotlight text The fabric of space: spin networks). These formulations fully respect the background independence of Einstein’s general relativity.

Further Information