How many different kinds of black holes are there?

Once they have settled down, there are actually only very few different kinds of black hole – find out which, and how black holes shed other distinguishing marks.

An article by Piotr Chrusciel

The collapse of a body to form a black hole can be a very dynamic and complicated event. But once the dust has settled, the evolution has played itself out and the system has calmed down, how many different types of black holes are there?

How many kinds of black hole?

This question is addressed by the so-called “black hole uniqueness theorems”. Even if we restrict ourselves to the matter and interactions ordinarily considered in astrophysics (for which the only long-range interactions are electromagnetism and gravity), leaving more exotic possibilities aside, there could be lots of different black holes. In fact, one might think there could be as many different black holes as there are ways of producing them: The collapse of an object shaped like a cube could lead to a cube-shaped black hole, the collapse of a cigar-shaped or disc-shaped lump of matter to black hole cigars or disc-shaped black holes, and so forth, as sketched here:

Objects of different shape collapsing to black holes of different shape?

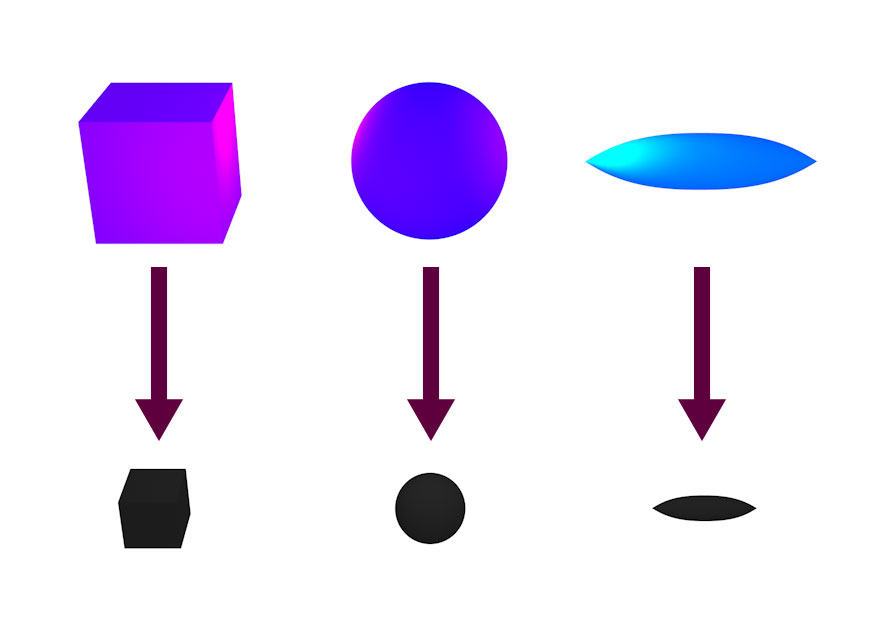
But as it turns out, in the long run, black holes are much more simple. They can be completely described in terms of a few parameters: the total energy, angular momentum (roughly, “how fast the black hole rotates”) and (of less interest to astrophysics), electric charge of the black hole. Once these parameters are chosen, the model universe – the way that spacetime geometry is distorted around the black hole – is determined completely. Sphere, cube or cigar – they all collapse to form a very simple kind of black hole that retains no traces of the original shape:

Objects of different shape collapsing to black holes of similar shape

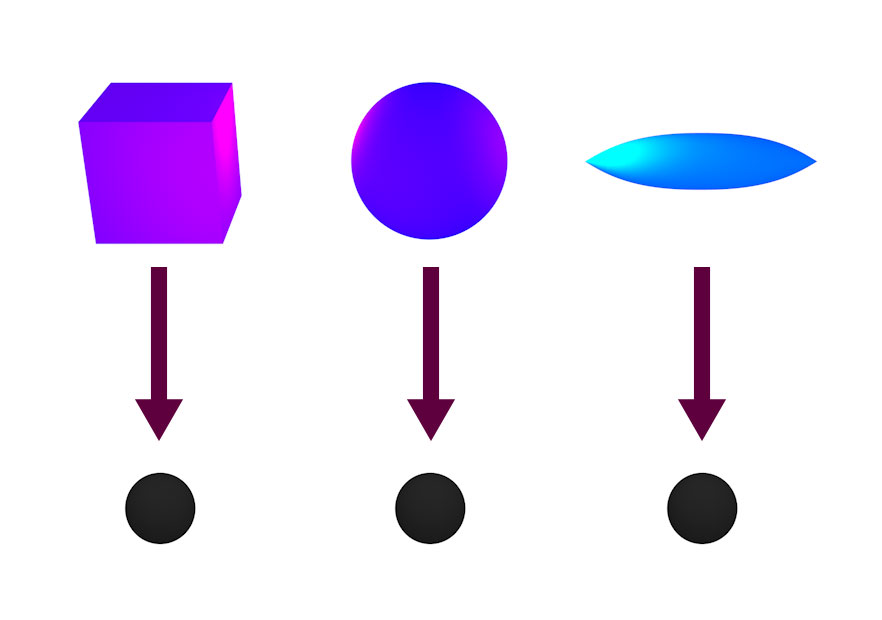
This striking property of black holes has been popularized as “the no-hair theorem” by John Wheeler (with the term itself invented by an anonymous member of the audience of one of Wheeler’s lectures). The name alludes to the fact that only very few parameters are needed to describe those solutions – apart from the values of those parameters, black holes have no distinguishing characteristics (no “hair styles”).

How do black holes lose their hair?

So how do black holes become simple? How does a cigar-shaped object that collapses to form a black hole lose all its cigar-like properties? In general relativity, there is a natural mechanism for simplifying the complicated geometric structure of certain regions of space: The emission of gravitational waves can carry away complicated features, with the waves either escaping into deep space or being swallowed by the black hole.

While a wide variety of features can be “radiated away” in this manner, there are exceptions. For instance, electric charge cannot be radiated away at all since neither the gravitational nor electromagnetic radiation carries such charge. More subtle restrictions apply to how much of the total mass or total angular momentum can be carried off (quantities for which there are so-called conservation laws).

All in all, the gravitational waves obey a rule set down in T. H. White’s novel The Once and Future King for a fictional society of ants: “Everything that is not forbidden is compulsory”. The gravitational field radiates away everything that can be radiated away. In consequence, the end state is uniquely characterised by a bare minimum of quantities: The final energy (equivalently, the final mass), and angular momentum, as well as possibly electric charge, with no additional features.

A gallery of simple black holes

So what are the different types of simple black holes that are stationary, in other words: that have settled down into an unchanging final state?

Let us start with vacuum black holes – black holes surrounded by space that is completely empty of all matter.

The simplest stationary vacuum black holes are the spherically symmetric ones. In fact, a theorem by J. T. Jebsen from 1921 (independently re-discovered by George D. Birkhoff in 1923 and nowadays named after him) shows that all spherically symmetric black holes are stationary, and they all belong to a family of solutions discovered by Karl Schwarzschild as early as 1916. The members of this family are distinguished by different values of a single positive parameter m. The value of m corresponds to the mass of the black hole, schematically:

Schematic depiction of Schwarzschild black hole characterized by mass m

The Schwarzschild solution is a special case of a family discovered by Roy Kerr in 1963, describing the most general stationary vacuum black hole. Such black holes are characterized by their mass m and angular momentum J, schematically:

Schematic depiction of Kerr black hole characterized by mass m and angular momentum J

If we take a Kerr black hole and set its angular momentum parameter to zero – corresponding to a black hole that does not rotate at all – the result is once more a Schwarzschild black hole.

The proof that these are indeed all vacuum black holes has a long history, initiated by Werner Israel in 1967, with contributions by Brandon Carter, Stephen Hawking and several other researchers.

Next, we can look at black holes that carry electric charge. These are not vacuum black holes, since the surrounding space is now filled with an electric field. In the context of general relativity, such fields are a form of matter – the associated energy is a source of gravity.

Stationary, electrically charged black holes are described by a a family of solutions which was found independently by Roy Kerr and Ted Newman in 1963. Black holes of this type are characterized by their mass m, angular momentum J, and electric charge Q:

Schematic depiction of Kerr-Newman black hole characterized by mass m, angular momentum J and electric charge Q

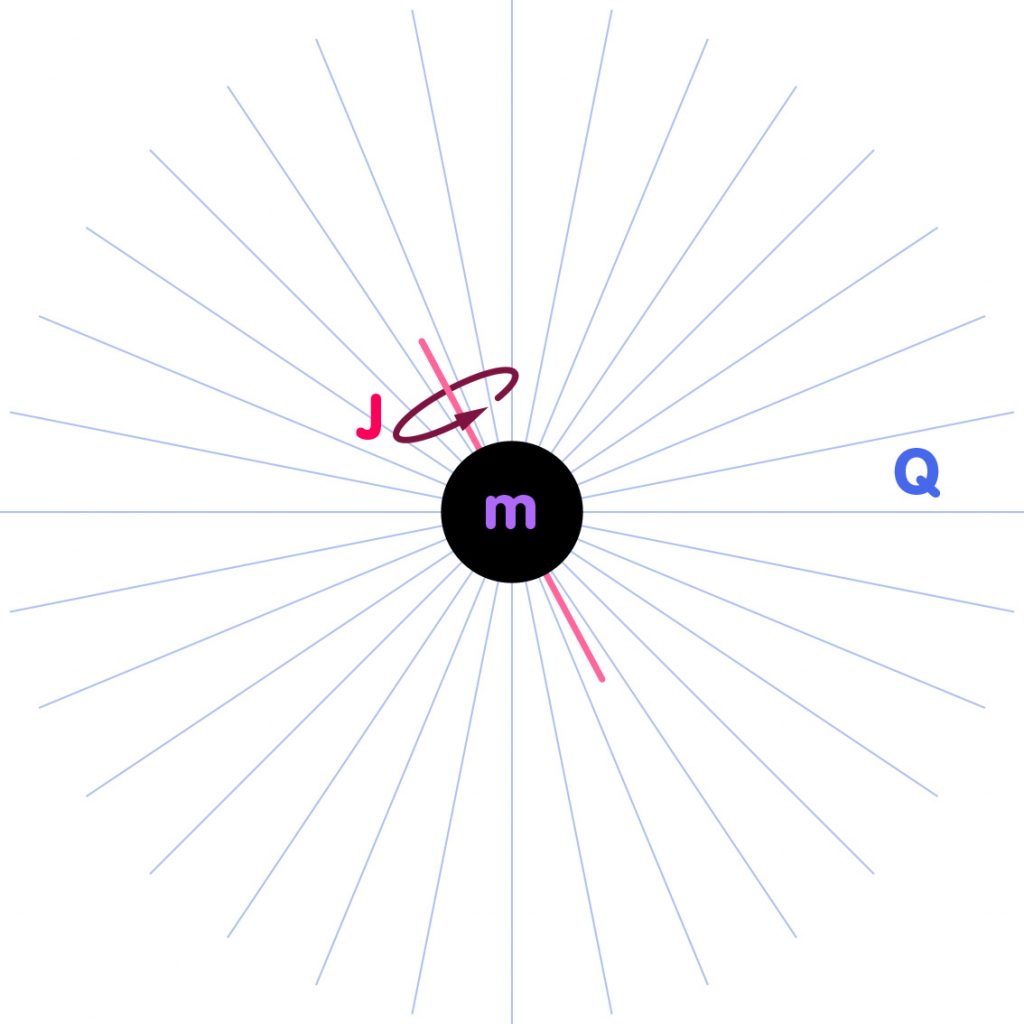
The uniqueness proofs have been extended to cover these solutions – and there we have them, the distinguishing features of black holes: mass, angular momentum, and electric charge.

Back to astrophysics

Strictly speaking, the uniqueness theorems describe lonely black holes that have had an infinitely long time to settle down into a stable, truly stationary state. But at least approximatively, they should apply to more realistic situations as well, which differ from the simple model situations in two respects: First of all, the black hole in question is not an isolated object in an otherwise empty universe, but merely an isolated object in the depths of space. Secondly, it has not had an infinitely long time to settle down, but merely a long time compared to what it takes to “shed its hair” in the form of gravitational waves.

This is good news: Astrophysicists can expect even realistic black holes to be described by the well-known Kerr solution, provided that enough time has passed for them to radiate away any distinguishing features. And for the researchers hunting for gravitational waves, black holes “shedding their hair” promise attractive sources.

Further Information

Relativistic background information for this Spotlight topic can be found in Elementary Einstein, in particular in the chapter Black holes & Co..

A related question of uniqueness versus variety – how many different kinds of empty, stationary model universes Einstein’s general relativity theory permits – is explored in the spotlight text The many ways of building an empty, unchanging model universe. Further related Spotlights on relativity can be found in the category Black holes & Co.. A concise technical introduction to the “no hair theory” can be found in the e-print gr-qc/0502041.

Colophon

Piotr Chrusciel

is a professor of gravitational physics at the University of Vienna. His research covers a wide range of mathematical topics related to Einstein’s equations.

Citation

Cite this article as:
Piotr Chrusciel, “How many different kinds of black holes are there?” in: Einstein Online Band 04 (2010), 02-1013

Definitioner

waves (wave)

In a general sense: any travelling pattern, whether or not it involves matter being transported as well. Simple examples are water-waves - wave crests and troughs travelling over a water surface, and a Mexican wave in a football stadium, with fans alternately standing up and sitting down - the pattern moves throught the stadium, not the fans themselves.

An especially simple form for a wave is a sinus wave, a regular pattern of wave crests and troughs.

uniqueness

Given a set of physical laws, one interesting class of question is aimed at finding out the variety of situations those laws allow. For example, is there only a single kind of rotating black hole, or do the laws of general relativity admit an infinite variety of such objects? Theorems addressing this kind of question are generally known as uniqueness theorems - in their purest form, they state that, given a certain set of physical laws and a certain set of additional conditions, there is no more than one configuration of spacetime and matter that fits the bill. In general relativity, the most famous such theorems are the black hole uniqueness theorems. They are explored in the spotlight text How many different kinds of black hole are there? A different aspect of the question of uniqueness is addressed in the spotlight text The many ways of building an empty, unchanging universe.

uniqueness theorems (uniqueness)

time

It is a fact of life that not all events in our universe happen concurrently - instead, there is a certain order. Defining a time coordinate or defining time, the way physicists do it, is to define a prescription to associate with each event a number so as to reflect that order - if event B happens after event A, then the number associated with B should be larger than that associated with A. The first step of this definition is to construct a clock: Choose a simple process that repeats regularly. (What is "regular"? Luckily, in our universe, all elementary processes such as a swinging pendulum, the oscillations of atoms or of electronic circuits lead to the same concept of regularity.) As a second step, install a counter: A mechanism that, with every repetition of the chosen process, raises the count by one. With this definition, one can at least assign a time (the numerical value of the counter) to events happening at location of the clock. For events at different locations, an additional definition is necessary: One needs to define simultaneity. After all, the statement that some far-away event A happens at 12 o'clock is the same as saying that event A and "our clock counter shows 12:00:00" are simultaneous. The how and why of defining simultaneity - a centre-piece of Einstein's special theory of relativity - are described in the spotlight topic Defining "now". With all these preparations, physicists can, in principle, assign a time coordinate value ("a time") to any possible events, and describes how fast or how slow processes happen, compared to that time coordinate.

stationary

Roughly speaking, in relativity, a situation or a spacetime is stationary if there is no change over time. To be more precise, one has to take into account that, in general relativity, time can be defined in many different ways, all of them equally valid for formulating the laws of physics. This leads to a modified definition: A situation or a spacetime is stationary if it is possible to define time in a way so that there is no change of its properties over time - if you follow the properties of a given region of space over time, they will not change.

space

In a strict sense: Space as we know it from everyday life: the totality of all locations in which objects can sit, with three dimensions. In a more general sense used by mathematicians, all kinds of sets of points are spaces - a line for instance, which has but a single dimension, or a two-dimensional surface, but also higher-dimensional spaces. Also, in such more general spaces, geometry can be different from the standard Euclidean geometry taught in high schools - such spaces can be curved.

spacetime

Already in special relativity, observers in motion relative to each other will not, in general, agree as to whether two events happen simultaneously, or as to how great is the distance between two objects. They do, however, agree as to what events there are, although not to when and where they happen. This observer-independent totality of all events is called spacetime. How spacetime is split into space and time can differ from observer to observer. Every-day space has three dimensions. Adding time adds another dimension - spacetime has four dimensions, all in all. We are used to the idea of a point in space - an object that has only one location and is completely defined once its space coordinates are given. In spacetime, a spacetime point is an object defined completely once its space coordinates and its time coordinate are given - which makes a spacetime point nothing but an elementary event. The idea of spacetime is, in addition to its role in special relativity, a building block of general relativity. Analogous to how a plane is flat, but the surface of a sphere is curved, in general relativity, curved or distorted versions of the simple, flat spacetime of special relativity play a role. Spacetime curvature, in general relativity, is intimately connected with gravity. For an introduction to the basics of both theories of relativity, check out the chapters Special relativity and General relativity in Elementary Einstein. Sometimes, it can be helpful to view spacetime in analogy to ordinary space - such analogies are explored in the spotlight topics Time dilation on the road (for time dilation) and Twins on the road (for the twin effect).

solution

In the context of general relativity: a solution or, more precisely, a solution of the Einstein equations is a model universe that follows the law of gravity as prescribed by general relativity. See also exact solution.

Schwarzschild solution

See Schwarzschild black hole

Schwarzschild black hole

A solution of Einstein's equations found by Karl Schwarzschild in 1916, which corresponds to a model universe that contains a single, spherically symmetric black hole. More precisely, the Schwarzschild solution is a whole family of solutions: Schwarzschild's formulae contain a free parameter m corresponding to the mass of the black hole. To each concrete value of m corresponds one specific solution to Einstein's equations, a spacetime containing a spherically symmetric black hole of mass m. The Schwarzschild solution is of practical importance as the outlying regions of the corresponding model universe describe the spacetime distortion around all kinds of objects that are spherically symmetric, or nearly so, such as the sun or the earth (cf. Birkhoff's theorem).

Schwarzschild solution (Schwarzschild black hole)

radiation

In a general sense: Collective name for all phenomena in which energy is transported through space in the form of waves or particles. In a more restricted sense, the word is often used synonymously with electromagnetic radiation.

no-hair theorem

See black hole uniqueness theorems.

momentum

Physical quantity that is equal to a body's mass times its velocity (in special relativity: its relativistic mass times its velocity). What makes momentum a useful quantity is that it is conserved - if several bodies interact, the sum of their momenta before and after the interaction is the same. Momentum is neither created nor destroyed, merely passed on from one body to another. In general relativity, momentum is one of a number of quantities (like mass and energy) that acts as a source of gravity.

matter

In general relativity: All contents of spacetime that contribute to its curvature: particles, dust, gases, fluids, electromagnetic and other fields. In particle physics: All elementary particles with half-integer spin, such as electrons and quarks, as well as their composites such as protons and neutrons, in contrast with force particles.

mass

In classical physics, mass plays a triple role. First of all, it is a measure for how easy it is to influence the motion of a body. Imagine that you're drifting in emtpy space. Drifting by are an elephant and a mouse, and you give each of them a push of equal strength. The fact that the mouse abruptly changes its path, while the elephant's course is as good as unaltered, is a sure sign that the mass (or, in the language of physics, the inertia or inertial mass) of the elephant is much greater than that of the mouse. Secondly, mass is a measure of how many atoms there are in a body, and of what type they are. All atoms of one and the same type have the same mass, and adding up all those tiny component masses, the total mass of the body results. Thirdly, in Newton's theory of gravity, mass determines how strongly a body attracts other bodies via the gravitational force, and how strongly these bodies attract it (in this sense, mass is the charge associated with the gravitational force). In special relativity, one can also define a mass that is a measure for a bodies resistance to changing its motion. However, the value of this relativistic mass depends on the relative motion of the body and the observer. The relativistic mass is the "m" in Einstein's famous E=mc² (cf. equivalence of mass and energy). The relativistic mass has a minimum for an observer that is at rest relative to the body in question. This value is the so-called rest mass of the body, and when particle physicists talk of mass, this is usually what they mean. Just as in classical physics, the rest mass is a kind of measure for how much matter the body is made up of - with one caveat: For composite bodies, the energies associated with the forces holding the body together contribute to the total mass, as well (another consequence of the equivalence of mass and energy). In general relativity, mass still plays a role as a source of gravity; however, it has been joined by physical quantities such as energy, momentum and pressure.

lead

Chemical element with the symbol Pb; each lead nucleus contains 82 protons. Lead nuclei, stripped of their electrons, are among the types of heavy ions which are brought into collision in particle accelerators such as the Relativistic Heavy Ion Collider in order to recreate the state of matter in the early universe shortly after the big bang.

Kerr black hole

The simplest kind of a rotating black hole: a model universe containing a single rotating black hole and nothing else. This solution to Einstein's equations was found by Roy Kerr in 1963.

Kerr solution (Kerr black hole)

The simplest kind of a rotating black hole: a model universe containing a single rotating black hole and nothing else. This solution to Einstein's equations was found by Roy Kerr in 1963.

gravity

See gravitation

gravitational waves

Distortions of space geometry that propagate through space with the speed of light, analogous to ripples on the surface of a pond propagating as water waves. For more informations about gravitational waves, please consult the chapter Gravitational waves of Elementary Einstein. Selected aspects of gravitational wave physics are described in the category Gravitational waves of our Spotlights on relativity.

gravitational field

The totality of all gravitational influences that one or more massive objects can exert on bodies in their vicinity.

More precisely: At every location in space, the gravitational field is defined as the acceleration that a small test particle present at that location would feel due to the gravitational forces of the masses around it.

gravity (gravitation)

In classical physics: An action-at-a-distance force by which all bodies that possess mass attract each other (see Newtonian theory of gravity), synonym: gravitational force. In Einstein's general theory of relativity: The fact that matter that possesses mass, energy, pressure or similar properties distorts spacetime, and that this distortion in turn influences whatever matter might be present. An introduction to the basic ideas of general relativity is provided by the section General relativity of Elementary Einstein. More information about the nature of gravity in general relativity can be found in the spotlight text Gravity: From weightlessness to curvature.

geometry

That part of mathematics concerning itself with surfaces or more general spaces as well as objects defined on such spaces, such as points or lines as well as the objects constructable from points and lines, such as triangles.

general relativity (general theory of relativity)

Albert Einstein's theory of gravity; a generalization of his special theory of relativity. For information about the concepts and applications of this theory, we recommend the chapter general relativity of our introductory section Elementary Einstein. Further information about many different aspects of general relativity and its applications can be found in our section Spotlights on relativity.

field

A field describes how a physical quantity is distributed in space and time. For instance, the area where electric forces act on a test particle is subject to an electric field. Or the gravitational forces which act on the mass of a test body define a gravitational field. In general a field contains energy, occupies space and can change over time.

event

In the context of special or general relativity: Anything that is defined by a single point in time and a single point in space, i.e. something that happens at a definite time at a definite location. Synonym: spacetime point.

energy

Physical quantity with the special property that, in physical processes, energy is neither destroyed nor created, simply transformed from one form of energy to another. Some of the different forms of energy that are defined separately are kinetic energy, thermal energy and the energy carried by electromagnetic radiation. Processes that transform one form of energy into another take place in all machines we use in everyday life, from the engine of a subway train (electrical energy into kinetic energy of the train) to an electric blanket (electrical energy into thermal energy). One important consequence of special relativity is that energy and mass are completely equivalent - two different ways to define what is, on closer inspection, one and the same physical quantity. See the keyword equivalence between mass and energy.

electromagnetism

Electromagnetism is the totality of all phenomena associated with the presence of electric charges, such as the electric force, magnetic force or electromagnetic waves. The basic laws of electromagnetism are Maxwell's equations. In the context of special relativity it becomes clear that electric and magnetic forces are relative - which one of them is active in a given situation depends on the observer. Say that, from the point of view of one observer, the attractive force between an electric conductor and a moving charged particle is purely electric in nature - nevertheless, for a moving observer at rest with respect to that particle, it is purely magnetic. Just as, in special relativity, it makes sense to talk of spacetime as a whole, seeing that it depends on the observer how that spacetime is then split into space and time, it makes sense to talk collectively about "the electromagnetic force", leaving it to the different observers to split this electromagnetic force into electric or magnetic parts.

electromagnetic radiation

Electromagnetic influences (in the language of physics: electric and magnetic field) which, even with no electric charges present, are locked in a state of mutual excitation so that they form a wave that propagates through space. As this wave transports energy, it is, by the usual physics definition, a form of radiation, called electromagnetic radiation. Depending on frequency, there are special names for different types of electromagnetic radiation; going from lower to higher frequencies: radio waves, microwaves, infrared radiation, visible light, ultraviolet radiation, X-rays and gamma rays. In the context of quantum theory, it turns out that electromagnetic radiation consists of tiny energy packets, called light particles or photons.

electric field

The electric force is a force by which electric charges act on each other; the electric field is the associated field.

Electric fields cannot be understood separate from magnetic fields - their complete description is only possible within the more general context of electromagnetism.

In the simplest case, namely in situations that do not change over time, the electric force is the so-called electrostatic force.

electric charge

The charge associated with electromagnetism: a property of objects that determines the strength of the electric force with which they act on other charged objects, and the strength of electric forces by which other such objects act on them. Moving electric charges produce a magnetic field, and are influenced by such fields.

conservation laws

Some of the most important quantities in physics are conserved : What they measure can neither be created nor destroyed, and their total sum is constant over time. Such statements of constancy over time are called conservation laws. The most important conserved quantity is energy. Energy can neither be created from nothing nor simply vanish. If the energy contained in a system increases, it must be because energy has been transported into the system (and there is now less energy outside the system); if the energy decreases, it must be because energy has been transferred from the system (and there is now more energy outside). Another important class of conserved quantities are charges, for instance electric charge. A further conserved quantity in mechanics is angular momentum; a quantity associated with a body's rotation.

charge

A measure of the strength of a force (action-at-a-distance) originating from a body, and of how susceptible it is to being influenced by other bodies via the same force. The most famous example is electric charge: Electrically charged bodies act on other electrically charged bodies via an electric force whose strength is proportional to the electric charges of the bodies involved. It is a characteristic property of charges that they are conserved; they can neither be created from nothing nor simply disappear. For instance, when a positron with electric charge +1 (in suitable units) and an electron with electric charge -1 annihilate to give electromagnetic radiation, overall charge conservation is satisfied: Before the annihilation, the sum of the electric charges was 1+(-1)=0, and afterwards, when there is only uncharged electromagnetic radiation left, it is also zero. In the context of particle physics, there are more abstract charges not directly connected with forces and interaction, but subject to similar conservation laws.

black hole

Region in space where a sufficient amount of mass is concentrated so that it forms a gravitational prison - a region into which matter or light can enter from the outside, but from which nothing that has once entered can ever leave. Basic information on this key phenomenon of Einstein's general theory of relativity can be found in the chapter Black holes & Co. of Elementary Einstein. Selected aspects of the physics of black holes and neutron stars are described in the category Black holes & Co. of our Spotlights on relativity. In Einstein's theory, black holes are truly black, due to the fact that no radiation or light can ever escape them. Once quantum theory is taken into account, this assumption no longer holds - on the contrary, it seems as if black holes should emit so-called Hawking radiation. However, for astrophysical black holes (that typically have more or even much more than one solar mass), that radiation would be undetectable, even if we could transport today's most sensitive sensors into the immediate vicinity of the black hole.

black holes (black hole)

black hole uniqueness theorems

Theorems proved in the context of general relativity that answer the question: How many different kinds of black holes are there? If that question is restricted to stationary black holes (namely black holes that have settled down and do not change over time), then the answer is: Surprisingly few. Once you know a stationary black hole's mass, angular momentum (roughly speaking, how fast it rotates) and electric charge, its properties are determined completely. More information can be found in the spotlight text How many different kinds of black hole are there?

angular momentum

A conserved physical quantity associated with the rotation of an object. In classical physics, the contribution of each part of a body to the body's total angular momentum is the part's mass times its distance from the axis of rotation, times the part's speed component which points in the direction of rotation and is perpendicular to the axis of rotation. More information can be found in the spotlight text What figure-skaters, planets, and neutron stars have in common. In the context of general relativity, angular momentum is an interesting quantity in the physics of black holes. More information about this can be found in the spotlight text How many kinds of black holes are there?